1. Introduction
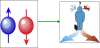
Physics has a long tradition studying bistable systems and where Ising model is well known for studying the interactions among spins [1,2]. The latest can reach value of 1 (spin up) or value of -1 (spin down) depending on the energy producing with their neighbours, or with those spins that are connected through the interaction lattice. Spins are acting mutually in pairs with energy, which has a specific value depending on the orientation of the two spins, the orientation can be the same or totally opposite. Generally speaking spins in their actions are very similar to actions of people that can be either cooperators or defectors (do not cooperate). From the left side of Figure 1 you can see two spins, one is blue and one is red. These two spins can be presented the same as person on the right side of Figure 1, who can behave as a cooperator, the blue arrow or as a defector, the dark red arrow.
Physicists therefore possess a rich array of both theoretical and experimental knowledge to enable effective and relevant analysis of bistable systems. Furthermore, physicists, together with other experts measure and develop models for all areas of environmental protection; including the managing climate change is one of the major problems currently facing the whole world. However, the environmental problem of climate change does not represent a major obstacle on the road to global climate solutions. The main obstacle is cooperation between countries and all other players, and so we come to a simple question: when will the participants willing to cooperate and when they act in a selfish way? The question leads to a situation where we can use a game called Prisoner’s dilemma [3].
The game allows players to reach mutual (total) benefit, but only if they decide to cooperate with each other. There is of course also the possibility that none of the participating players cooperate, whereby the individual gains increase, but this effect is short-lived. In our case, the players are people who represent the participating countries. This fact is somehow reassuring, since we humans compared to other mammals are masters of cooperation [4]. Mutual relations are the key in this whole process. The basic idea is that if I help you today, you will help me tomorrow. Research shows that this simple concept is the secret of success [5]. Physical models help us to better understand the impact of individual strategies, personal decisions and interaction networks/lattices between the various players on the climate change. Climate change is seen as phase transitions in the related bistable systems [6].
Prisoner's dilemma game is addressed through interaction lattices between individual players. We choose one player and one of his neighbors, while assuming that the neighbor is associated with him. Connections between individual players form interaction lattices. Initially at time t=0, the player is determined either as cooperator ( Sx = C) or defector (Sy = D ) with the equal probability. Thus we have connected Prisoner’s dilemma to a spin system through Fermi function, which includes two strategies – cooperation and defection. Evolution of two strategies is performed by comparing players payoffs that have been produced when playing with their neighbours. Probability of adopting a certain strategy is shown by the following Fermi function [7]:
Where K quantifies the uncertainty in the strategy adoption process.
We assume that strategy of player x (Sx) is cooperation and strategy of player y (Sy) is defection. Probability of changing player’s y strategy by adopting strategy Sx, which means a change from D to C, depends on the difference between payoffs Py in Px. If Px < Py, then W → 0. If Px > Py, then W → 1. The latest we can very clearly see on Figure 2.
Following the standard dynamics of bistable spatially-extended models, we use Monte Carlo simulation and randomly select player x and one of its neighbors y. After calculating the payoffs and , player x tries to implement its strategy to player y in accordance with the probability W, K Є (0, ∞). K defines temperature as a free parameter, which in the context of Prisoner's dilemma game determines the level of irrational decisions [8] or the uncertainty associated with the acquisition of a certain strategy [7].
If the uncertainty parameter is small (K near 0), the probability of adopting strategy Sx by player y is smaller than it if the uncertainty parameter is high. Therefore, when we are dealing with high level of uncertainty, the probability of strategy adoption (in our case this means a player’s strategy with lower payoff) can change and we can face the situation, when this strategy is going to be adopted, what would not be a case in the circumstances with low level of uncertainty [9].
Environmental studies often use the Prisoner's dilemma game to analyze the behavior patterns of individual countries in the search for a global objective. All countries would benefit from a cleaner environment or reduced greenhouse gas emissions, but on the other hand, many countries remain inactive by measures for assuring sustainable development and would continue with environmental pollution [10].
2. Deterministic and Stochastic Environments
Deterministic environment is environment where everything is precisely defined and at the same time everything is very easy to predict (when K → 0), no information is ever lost. Such type of environment is shown on the left side of Figure 3. Stochastic environment is uncertain and unpredictable, information are not reliable (when K > 0). And this is shown on the right side of Figure 3.
Stochastic environment as opposed to a deterministic environment is uncertain and unpredictable environment. At the same time it varies and the information is not reliable. The choice of strategies is more or less random. If someone in a stochastic environment shows a higher return, it does not mean that this is really true. Many real processes we encounter in everyday life show the properties of stochastic processes. For example, the demand for a product that we do not know in advance and the same time we introduce a random variable in the stochastic model. When K>0, we are dealing with a stochastic environment, but when K →∞ an evolution strategies is completely independent of Px and Py, and is in fact identical to the toss of the coin.
The most interesting is that the reality is somewhere in between, the environment is not completely deterministic, but also not completely stochastic. In the Prisoner's dilemma game, therefore, we are interested in, which is a critical b, wherein cooperators die out, where T = b [1,2]. At the same time we are considering that we are situated in the stochastic environment where K = 0.1.
As shown on the Figure 4, for cooperators to survive is optimal slightly stochastic environment, where the value of the parameter K = 0.1. By increasing the value of the parameter b, the share of cooperators decreases and at a certain value of b, which is called the critical value (bc), the cooperators die out. In a completely deterministic environment (K = 0), by the values of b that are greater than the critical value, defectors dominate.
If we go further with our analysis, we can see that cooperators can survive at K levels that are different from 0 and at values b that are higher than 1, in our case 1.04.
Cooperators create small groups called “clusters” that are distributed among entire spatial lattice, what can be very clearly seen in the middle panel. The clusters are the blue ones.
There is always an optimal level, where the fraction of cooperators is at highest and this indicates the existence of coherent resonance in the Prisoner’s dilemma. As we can see, at different values of parameter b, the share of cooperators is always highest at some intermediate value of parameter K.
Figure 5 shows that, despite the values of b > 1 cooperators can survive, which is contrary to theoretical forecast, which says that at these values only defectors should survive (Nash equilibrium) [11]. How do actually cooperators survive? As we have already mentioned that cooperators form small groups called "clusters" and those within these groups are protected from defectors and therefore the external cooperators protect the internal cooperators against the attacks of defectors.
In Figure 5, the left panel shows an almost deterministic environment (K → 0), the right panel shows a strong stochastic environment (based on the values of K > 1 comparable to K → ∞) and the middle panel means intermediate the environment between the first and second environment - mild stochastic environment. It follows that the medium-intensive stochastic environment is optimal for the evolution of cooperation.
On the other hand deterministic systems can show apparently random behavior, this behavior is known as deterministic chaos. Chaotic systems are extremely sensitive to small changes in the initial state, a response popularly referred to as the butterfly effect. Chaotic behavior is fully deterministic if you know the initial state in perfect detail, but any imprecision in the initial state, no matter how small, grows quickly (exponentially) with time. Climate is an example of how a chaotic system can conform to overall patterns. You may not be able to say what days it will snow five years from now, but you can still say what time period winter will be. What is very important is that chaotic systems are predictable for a while and then appear to become random. As it was pointed out chaos theory looks at the unpredictability of nature and tries to make sense of it [10].
3. Climate Dilemma
In the relations between countries often comes down to it that the interests of one country are in conflict with the interests of other countries. The most drastic example of such conflicting interests is a state of war.
Here endeavor to each affected site in order as soon as possible and in the most thorough way to destroy or at least temporarily eliminated all the forces of the opponent. Crossed interests tend to refer to conflict situations. Game theory is a theory of action precisely in such conflict situations [12]. In our Prisoner's dilemma game, instead of players we present each country. A very important fact is that, after the cooperation in the world of conflict situations is established, it is necessary to maintain the cooperation. Mutual cooperation remains stable if the future is relatively more important than the present.
Climate as a public good can quickly lead to misleading actions of individual countries, as these would like to contribute less at the expense of other countries and with such behavior they will create a social dilemma. If social dilemma is not resolved in an appropriate manner, we will soon be confronted with a so-called tragedy of commons [13]. The tragedy of commons, which in our case can be identify as a stabilizing the global climate, has been already introduced in 1968 by Hardin; as a metaphor for our inability to maintain public goods or commons, which we all can use free of charge [14]. Use of public goods does not create common (collective) benefits because usually individual interests are much different than the common interest. The findings of one study suggest a greater personal support to stabilize the climate if we leave individuals (in our case individual countries) to contribute more to the public good. Thus the transfer of information is crucial, because better informed participants contribute more frequently and more money for solving the global climate problem [15].
The main problem, therefore, is that the climate is a public good shared by all mankind. Individuals are not willing to invest in managing climate change, whereas the direct benefits that individual’s gain from investments are much smaller than the costs. The climate is therefore very sensitive and is faced with the tragedy of commons. If we want to achieve mutual cooperation between individuals, we must change the rules, for example where participants can punish or reward other participants, the investment remained at a high level. The main message is that if the behavior of an individual is associated with the public good the activities of an individual should be public - getting the reputation or good image [16].
Maintain global climate is the biggest game with public goods. It is the game that concerns all of us and we really cannot afford to lose it. We must learn how to cooperate at the global level, how to respect the needs of others and how to avoid wasteful lifestyle. Evolutionary dynamics are creative precisely because of the cooperation. Whenever the evolution has discovered something completely new (e.g. the first cell) also the cooperation has been included. Participation means that people pay the costs of others to obtain the benefits. Indirect reciprocity works through reputation. People who want to help have a good reputation and will often receive help than others who are not willing to help (so-called "free-riders"). Environmentally friendly behavior should be rewarded through special reputation marks that would have been treated as valuable signals. Environmentally harmful activities should be punished and stigmatized in a way to transmit the message that they are dangerous for our climate [17].
Each country is better off, if we all share the benefits of public goods. The climate certainly is a public good by which the issues of ownership and cost sharing are emerging. We must learn how to cooperate on a global scale and how to respect the opinions of other participants. An effective global climate agreement should allow the different behavior patterns of the participating countries, but it all has to be defined in the direction of long-term mutual cooperation [18].
The climate change is a global problem and therefore its solution needs to be built upon reciprocal cooperation, at the national as well at the international level. A temptation to contribute less and save money to induce others to contribute more will always be present.
The problem arising within this "climate game" is that the whole world is realizing that tackling the climate change will be very costly. It will most certainly require rather deep structural changes in energy supply and transportation systems, and what is even more important; it will also require changes in human behavior at the level of each individual. For a successful global climate agreement it therefore seems essential that different countries realized that everything is based on human relationships and the evolution of cooperation.
Developed countries, acting like clusters, can act as a safe haven also for other “potential” cooperators, and importantly, the hope that cooperation will remain viable. Uncertainty can thereby tip the balance in favor of cooperation. Climate targets cannot be reached unilaterally, but only at the level of global cooperation. Cooperation surely represents long-term stable strategy, which can contribute to designing successful and efficient long-term global climate agreement.
Countries behave very similar to selfish individuals and therefore Prisoner’s dilemma game can be used for analyzing behavior patterns of individual countries. Cooperation theory says that cooperation starts in very small groups “clusters” and is then gradually expanded. We need to emphasize that clustering is an essential feature that allows cooperators to survive. Moderate unpredictability might be an important agonist pushing towards a successful resolution of the climate change problem.
4. Challenges for the Future
International collective action is necessary for tackling the global climate problem and for moving into the direction of low-carbon economy, which is the only reasonable solution. Prisoner’s dilemma game is a suitable tool for searching a solution within climate problem. Unpredictability can offset the temptations to pollute, but it needs to be properly expressed, i.e. not facilitated by politics and other influences that tend to have a self-centered agenda. While the effects of climate change are still somewhat uncertain, the moderate unpredictability might be an important agonist pushing towards a successful resolution of climate dilemma.
Simultaneous progress in five key areas: increasing the energy efficiency; sustainable energy services available in developing countries; transfer of “clean” energy technologies; improvement in terms of sustainable transport; optimal use of economic instruments to reduce or limit greenhouse gas emissions.
Climate game therefore involves investing in the public good, but not for profit, but for the purpose of avoiding losses that would threaten the existence and functioning of future generations [17]. A global climate agreement will be reached only if the cooperation between the participating countries is successful. Countries are players who will participate in the climate game or not. Cooperation theory says that cooperation may start in very small groups of individuals although at the moment no other in the world is willing to cooperate [3].
In reality, the final result depends not only on the two players or two countries, but also on other factors, for example asymmetry (climate costs are not the same for all countries), bargaining and political power (usually different for the participating countries) and the factors that are stochastic in nature - the uncertainty and unpredictability of the information. Stochasticity is recently emerging as a potential factor of ensuring the cooperative behavior in the Prisoner's dilemma game, assuming compliance with a certain level of noise intensity as completely unpredictable factor [19].
The basic principle of all players in the global climate game should be to create a low-carbon global economy. Technological changes or innovations could be triggers for reducing the carbon dependency of all players or countries. Reducing carbon dependence can be achieved through direct emissions policies or through technology policies. Implementation measures for the first type of policy are the introduction of eco-taxes and duties, emissions trading scheme by setting the caps on the greenhouse gas emissions (GHG) and subsidies for reducing GHG emissions. The implementation measures for the second type of policies are subsidies for research and development into low-carbon technologies, the participation of the public sector in research and development into low-carbon technologies as well as rewards from the government for financing the environmentallyfriendly technologies.
It is important that, after cooperation established in our world, it can also be maintained [3]. Mutual cooperation remains stable if the future is relatively more important than the present. Each individual could be better off if all pay and also all share the benefits of the public good [20]. Our natural environment definitely belongs to the so-called public goods where ownership and cost sharing problems occur. External negative effects that occur by public goods may be offset by economic or social instruments. The economic instruments include environmental taxes, charges, trading with emissions allowances and credits, subsidies. The social instruments include specific arrangements between participants [21]. One of the most important agreements in the area of climate change that will shape our climate future is certainly a global climate agreement, which was adopted in Paris by 195 countries on 12 December 2015 [22].
Finally they also demonstrate the importance to coordinate and integrate products that have been developed separately from one another but have clear synergies, especially in the field of urban climate. It should be mentioned that the application presented in this study, is of demonstration character which implies that the drafting and assessment of the planning strategies need to be based in more detailed spatial and temporal calculations of the energy fluxes and the state of the thermal environment in the area under investigation.
5. Paris Agreement
The Paris Agreement is a bridge between today’s policies and climateneutrality before the end of the century. Participating governments have agreed [23,24]:
- a long-term goal of keeping the increase in global average temperature to well below 2°C above pre-industrial levels;
- to aim to limit the increase to 1.5°C above pre-industrial levels, recognizing that this would significantly reduce the risks and impacts of climate change;
- on the need for global emissions to peak as soon as possible, recognizing that this will take longer for developing countries;
- to undertake rapid reductions thereafter in accordance with the best available science.
Agreement also recognizes the importance of averting, minimizing and addressing loss and damage associated with the adverse effect of climate change. It also acknowledges the need to cooperate and enhance the understanding, action and support in different areas such as early warning systems, emergency preparedness and risk insurance. The agreement is due to enter into force in 2020.
6. Conclusion
No matter how two systems start together and no matter how similar are the initial conditions there are still some differences and exactly these small differences lead to a completely different future. For this reason, we can never know exactly what will be our future or the future of any system. Everything is in the hands and laws of chaos. On the other hand we should not forget the nature of social behaviour when dealing with climate dilemma. The principle of least effort, a concept advanced by the American linguist George Kingsley Zipf, indicates that people complete tasks by choosing the way of least effort among various options [25]. Such kind of behaviour we can also sense during the negotiation process for achieving the new global climate agreement. The core element in every negotiation is human being and human behaviour is highly complex with its unpredictability. The latest has been discussed by Stehlik [26] who has studied the impact of the chaos theory on the methane emissions. Thus this paper has focused on the deterministic and stochastic environments, the chaotic behaviour is not considered in detailed but it should not be neglected [27-30].
Looking again at Figure 5 the population is on the good way to achieve the middle panel, where the share of cooperators is at highest. At the moment we are in the phase where defectors still dominate but the Paris Agreement with its three key elements, ambition, commitment and solidarity, will achieve that the cooperators will start to dominate over defectors [31,32]. The European Union as a cluster in the role of the global leader in climate action has succeeded. Countries should strengthen their cooperation based on the following activities as sharing information, good practices, experiences and lessons learned.
Acknowledgments
Part of this paper was prepared in the framework of the project.